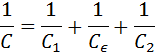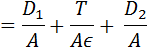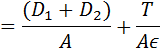Moving Dielectric

One of the most appealing things about the moving dielectric method of variable capacitance is that one of the constraints for the moving part is not required.  That is, the position of the dielectric between the two fixed metallic plates does not affect the capacitance.

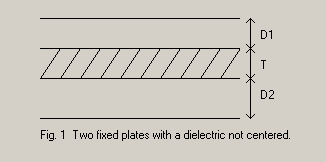
We assume the dielectric is of permittivity Є.  The three parts of the capacitor can be treated as three capacitors in series:

   ,  ,

where the natural unit of capacitance is one of length.

The total capacitance for capacitors in series is:

Here C depends only on the sum D₁ + D₂ and therefore not on where the dielectric is in-between the two plates! This is a great convenience since the constraint on that variable is released and the dielectric plate can be allowed to go where it wants in this dimension.

Now viewing from above if two plates are interposed by a dielectric in part of their area:

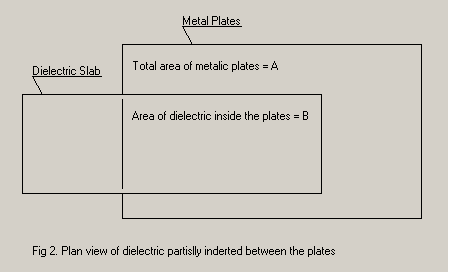
In the part of the plates outside the dielectric, the capacitance of the two plates is  (A-B)/(D₁ + D₂ + T), i.e. the area outside B divided by the total separation. The capacitance of the area B is B/(D₁ + D₂ + T/E).

The total capacitance is just the sum of the above since they are in parallel:

^-

The B term is the capacitance added by the introduction of the dielectric and for constant geometry and permittivity is just proportional to B. This is convenient since methods to measure the capacitance based on an RC time constant give a result which is linear in B.